First countable spaces without point-countable 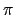 -bases
-bases
István Juhász,
Lajos Soukup and Zoltán Szentmiklóssy
We answer several questions of V. Tkacuk from [Point-countable 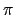 -bases in first countable and similar spaces,
Fund. Math. 186 (2005), pp. 55-69.] by showing that
-bases in first countable and similar spaces,
Fund. Math. 186 (2005), pp. 55-69.] by showing that
- there is a ZFC example of a first countable, 0-dimensional Hausdorff space
with no point-countable
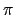 -base (in fact, the order of any
-base (in fact, the order of any
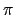 -base of the space is at least
-base of the space is at least
 );
);
- if there is a
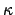 -Suslin line then there is a first
countable GO space of cardinality
-Suslin line then there is a first
countable GO space of cardinality 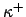 in which the order of
any
in which the order of
any 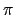 -base is at least
-base is at least 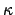 ;
;
- it is consistent to have a first
countable, hereditarily Lindelöf regular space having uncountable
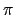 -weight and
-weight and 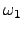 as a caliber (of course, such a space
cannot have a point-countable
as a caliber (of course, such a space
cannot have a point-countable 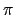 -base).
-base).
Key words and phrases:
2000 Mathematics Subject Classification:
Primary: , Secondary:
Downloading the paper