On the weak Freese-Nation property of
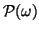
Sakaé Fuchino, Stefan Geschke and Lajos
Soukup
We study the consequences of the weak Freese-Nation property of
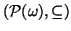 .
Under this assumption, we prove that most of the known cardinal
invariants including all of those appearing in Cichon's diagram take
the same value as in the corresponding Cohen model. Using this principle we
could also strengthen two results of W. Just about cardinal sequences of
superatomic Boolean algebras in a Cohen model. These results show that
the weak Freese-Nation property of
.
Under this assumption, we prove that most of the known cardinal
invariants including all of those appearing in Cichon's diagram take
the same value as in the corresponding Cohen model. Using this principle we
could also strengthen two results of W. Just about cardinal sequences of
superatomic Boolean algebras in a Cohen model. These results show that
the weak Freese-Nation property of
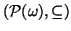 captures many of the
features of Cohen models and hence may be considered as a
principle axiomatizing a good portion of the combinatorics available in
Cohen models.
captures many of the
features of Cohen models and hence may be considered as a
principle axiomatizing a good portion of the combinatorics available in
Cohen models.
Downloading the paper